Impossible

There are numerous constructions that have been proven impossible to be constructed using compass and straightedge alone. These include angle trisection, cube doubling and circle quadrature. However, they can be constructed through approximations or even marked straightedges. The first example illustrates this.

Angle Trisection
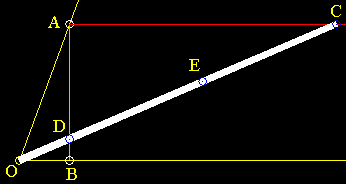
Given angle AOB, trisect it. This can be done using a marked ruler.
  1. Take a ruler and mark points D,E and C, so that DE=EC=AO.
  2. Draw line from point A (red) parallel to OB.
  3. Draw line from point A perpendicular to OB (cyan).
  4. Using your mared ruler, position it so that it crosses O, point D crosses the perpendicular line (cyan) and C crosses the parallel line (red).
  5. The ruler trisects angle AOB!

Circle Squaring

To construct a square equal in area of a given circle is impossible. However it can be approximated. The area of a given unit circle is p. Then a square of equal area will have a side equal to the squareroot of p. There is no construction for p, however, there is a very good approximation for it, known as Kochansky's Approximation.

Kochansky's Approximation

  1. Construct 4 collinear points A,B,C and D, such that AB = BC = CD = 1.
  2. Construct a line perpendicular to AD with points E and F, such that AE = EF = 1.
  3. Construct a line perpendicular to AF through point E. Find G on that line such that EG = 1.
  4. Draw a circle at E and G, each having radius EG. They cross each other at H.
  5. Draw a line through points EH.
  6. Draw a line perpendicular to AF through point F. This line intersects EH at I.
  7. Connect points I and D. ID is approximately p.
To complete the construction, take the squareroot of p.

© Apr.7 2003 Robin Hu. All Rights Reserved.